|
The story of milli and micro
Powers of ten and unit prefixes
-- 11 October, 2001
Background
Struggles with units of measure have a long and somewhat
sordid history. People have been measuring things (at least
coarsely) since we learned how to talk. We have needed the
assistance of units of measure in order to communicate
distances (to sources of food or water), time (to plant and
harvest crops), and weight (in trade) among others.
As societies grew (bearing in mind that communication was
once slow and tedious), different people in different places
invented units of measure in forms and scales on an "ad hoc"
basis as convenient to their daily lives. Measures of
length, time, and weight often differed from town to town,
much less country to country. Meanwhile, different and odd
units were invented for measuring things small (e.g.,
inches) vs. large (e.g., miles, furlongs). While being
convenient for everyday life in simpler times, these
traditional units of measurement made life messy when
society became more industrialized. Traditional units
complicated conversion between measures of big things and
measures of small things.
As communications improved, and political units grew
larger, commonality of measures improved -- but this clutter
didn't really start clearing up in earnest until the 18th
century. In 1790, French investigations into reform of
weights and measures resulted in the development of the
world's first "metric system." Over the years, this was
improved and refined until in 1960 the "modernized metric
system" (a.k.a., SI for Système International
d'Unités) made its formal appearance. SI units
brought a new, cleaner way of dealing with units of
measurement and scale size. Base units for various measures
would be interrelated in a simple way, and scaling of almost
any unit would be done via a decimal (powers of ten) scheme
(only time and angles were left out of this -- but that's
another long story...). Note that while some countries have
still not completely converted to SI units for daily life,
SI is still the international standard for scientific
endeavors.
In spite of the advantages of decimal units, the SI
system would be clumsy in practical use if it went no
further than this. For example, 1 Farad (the base unit of
capacitance) is big, really too big to be convenient for
most purposes. A common size for capacitors
in BEAM
robots is 2.2 x 10-7
Farad; a common size for capacitors
in many other applications is 1 x
10-12 Farad. A basic
decimal system would lead to mathematical messiness by
forcing you to carry lots of digits and powers of ten around
in various domains of study.
Fortunately the SI system accommodates both the desire
for simpler math (via decimal units), as well as the need
for measures scaled appropriately to a given task. This is
done via unit prefixes based on powers of ten. Rather than
carry the full numeric notation for a given power of ten, a
"shorthand" notation is provided for units related to their
base unit by powers of ten. Using this shorthand, then, you
can describe small things via millimeters (thousandths' of a
meter, mm), or large things via kilometers (thousands of
meters, km), and not have to deal with difficult conversions
between them.
|
Multiple
|
Prefix
|
Symbol
|
|
|
yotta
|
Y
|
|
|
zetta
|
Z
|
|
|
exa
|
E
|
|
|
peta
|
P
|
|
|
tera
|
T
|
|
|
giga
|
G
|
|
|
mega
|
M
|
|
|
kilo
|
k
|
|
|
hecto
|
h
|
|
|
deka
|
da
|
|
|
deci
centi
|
d
c
|
|
|
milli
|
m
|
|
|
micro
|
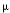 (u)
(u)
|
|
|
nano
|
n
|
|
|
pico
|
p
|
|
|
femto
|
f
|
|
|
atto
|
a
|
|
|
zepto
|
z
|
|
|
yocto
|
y
|
Returning to our capacitance example, BEAMers
can deal with capacitors
sized in microfarads, and other electronics designers can
work with capacitors
sized in picofarads. Mind the powers of ten associated with
this shorthand, and the math all works out cleanly.
At the same time, the SI system provides both a prefix if
you wish to spell out a unit name (e.g., microfarad),
and a symbol if you prefer to use the unit's symbol (e.g.,
uF).
|